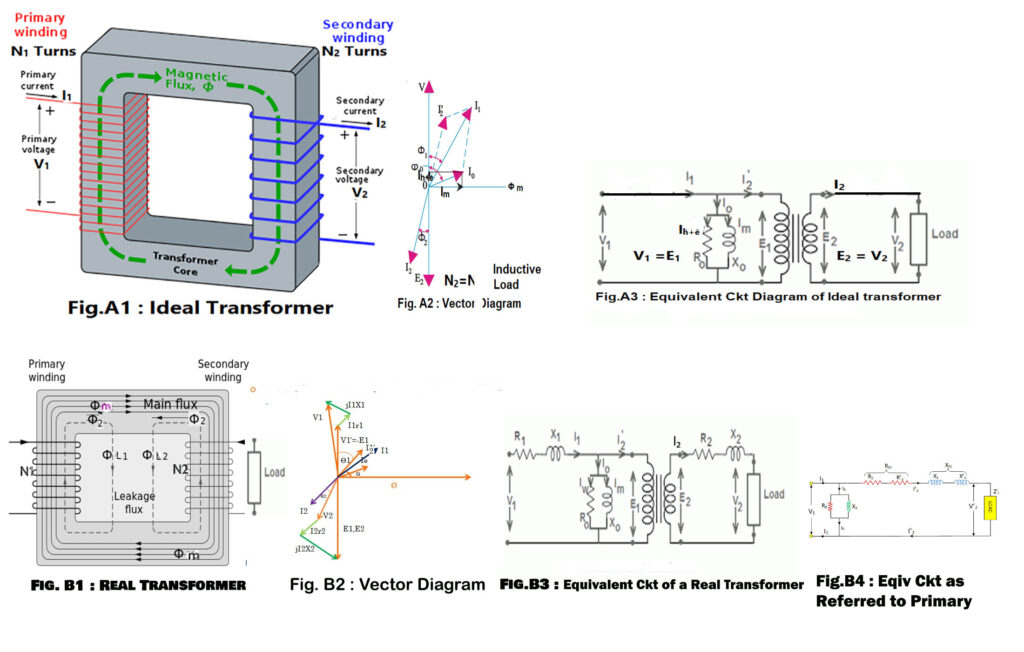
Description :
A. Equivalent Circuit of an Ideal Transformer:
Fig. A1 shows the schematic diagram of a single-phase transformer. When the input voltage V1 is applied to the primary winding (N1 turns) under no-load condition, no-load current (Io) & a flux (Φm) is produced in the primary ckt & the magnetic core respectively. No-load primary current Io has two parts – loss component, Ih+e & it is called hysteresis and eddy current which is in phase with V1 & other part is magnetizing current Im which is 90o out of phase with V1. Magnetizing component Im has no contribution to any loss but it’s responsible for generating flux.
Fig. A2 shows the vector diagram under inductive load where current I2 lag voltage E2.
Ih+e= Io cosθ0
Im = Io sinθ0
Io = √ ( Ih+e ^2 + Im ^2 )
E1= Back emf induced in the primary winding.
E2= Back emf induced in the secondary winding.
V2 = Secondary terminal voltage
At no load, the only loss is the iron loss due to hysteresis & eddy current loss. In the case of an ideal transformer, under also load condition winding resistance & leakage reactance are eliminated considering the infinite permeability of the core.
Fig. A3 shows the equivalent ckt of a ideal transformer where V1 = E1 & V2 = E2 .
B. Equivalent Circuit of a Real Transformer:
Fig. B1 shows the schematic diagram of a single-phase real transformer. When the input voltage V1 is applied to the primary winding (N1 turns), apart from the generation of no-load current(Io) & flux (Φ m ) in the primary side, loaded secondary side (N2 turns) will set up a current I2 (Fig. B3) whose value depends on the type of the load & secondary voltage. Current I2 in phase with secondary voltage V2 for resistive load, lag V2 for inductive load & I2 lead V2 in case of capacitive load. Secondary current will generate its magnetomotive force, mmf & a momentary flux Φ 2 which direction is opposite to the main flux Φ m .
Therefore, this (Φ 2 ) flux weakens the main flux which causes some momentary gain of V1 over E1 (back emf induced in the primary side) & more current ( in addition to Io ) flows through the primary circuit. Fig.B2 shows the vector diagram where I2 ‘ is the additional primary current flows in the primary winding due to I2 along with no load current Io . This additional primary current I2 ‘ further produces a flux Φ2 ‘ whose direction is opposite to Φ 2 but in the same direction of main flux Φ m . As the Φ 2 & Φ2 ‘ are the same magnitude, they cancel each other & the main flux will remain the same.
Here , Φ 2 = Φ2 ‘
⮞ N2 I2 = N1 I2 ‘
⮞ I2 ‘ = (N2/N1) I2 ————— (1)
Why the no-load loss or iron loss always same?
Since the flux(Φ 2) produced by the secondary current & opposite flux( Φ2 ‘) of the same magnitude produced by the primary current (as discussed above) cancel each other & only the main flux (Φ m , produced at no load ) persists, we can conclude that no-load loss or iron loss is constant at all load ( full load, no-load, half load, etc) conditions.
Winding Resistance :
Actually, primary winding & secondary winding have some resistances & current flows through the windings make voltage drop (IR) and some losses. Therefore, primary induced back-emf E1 is less than V1 by an amount I1R1 whereas secondary induced back emf E2 is greater than V2 by an amount I2*R2 .
V1 = E1 + I1R1 & V2= E2 – I2R2
Where R1 =Primary resistance, R2 =Secondary resistance
We can refer secondary resistance to primary & vice versa in the following way
Cu loss in secondary side = I2^2*R2 & if R2’ is the equivalent resistance in the primary then equivalent Cu loss = (N2/N1)^2 *I2^2 *R2’ [ from Eqn (1) ]
⮞(N2/N1)^2 *I2^2 *R2’ = I2^ 2 *R2
⮞ R2’ = (N1/N2)^2 *R2, So this is equivalent secondary resistance as referred to the primary.
Similarly, equivalent primary resistance as referred to secondary is
⮞ R1’ = (N2/N1)^2 *R1
Leakage Reactance:
All the flux produced in the primary winding does not link the secondary winding. Apart from main flux Φ m , a part of magnetic flux (Φ L1 ) in the primary winding goes through the air path rather than magnetic core as the real iron core has not infinite permeability. This leakage flux is known as primary leakage flux (Φ L1 ) that does not link the secondary turns & proportional to primary amp-turns. The flux (Φ L1 ) is in phase with current I1 & induces a self-induced emf eL1.
Similarly, a leakage flux (Φ L2 ) is produced in the secondary winding due to secondary amp-turns which is linked with secondary winding only, not with the primary as it completes the circuit through the air path. The flux (Φ L2 ) is in phase with secondary current I2 & induces a self-induced emf eL2.
Leakage fluxes are negligible in case of no-load & small load. But high load means high currents flow through the primary & secondary sides & increase the leakage flux. Since the leakage fluxes/ self-induced emfs do not link other winding, they don’t contribute to an energy transfer from primary to secondary winding.
Therefore, it is equivalent to an inductive coil in series with each winding so that voltage drop in the series coil is equal to that generated by that leakage flux.
Primary leakage reactance , X1 = eL1 /I1
Secondary leakage reactance , X2 = eL2 /I2
Similar to resistance, X2’ = (N1/N2)^2 *X2 ⮞ equivalent secondary reactance as referred to the primary.
And equivalent primary reactance as referred to secondary is
⮞ X1’ = (N2/N1)^2 *X1
Resistance & Leakage Reactance on Real Transformer / Vector Diagram :
Fig.B2 & Fig.B3 show the vector diagram and equivalent circuit of a real transformer respectively.
Primary Side : V1 = E1 + I1R1 + I1X1
Secondary Side: V2 = E2 – I2R2 – I2X2
Z1= √ ( R1^ 2 + X1^ 2 ) & Z2= √ ( R2 ^2 + X2^ 2 )
If secondary resistance, R2 & leakage reactance, X2 are transferred to primary winding ( Fig.B4)
X01 = X1 + X2’ & R01 = R1 + R2’
Z01 =√ ( R01^ 2 + Xo1^ 2 )
If primary resistance, R1 & leakage reactance, X1 are transferred to the secondary winding
X02 = X2 + X1’ & R02 = R2 + R1’
Z02 =√ ( R02 ^2 + Xo2^ 2 )